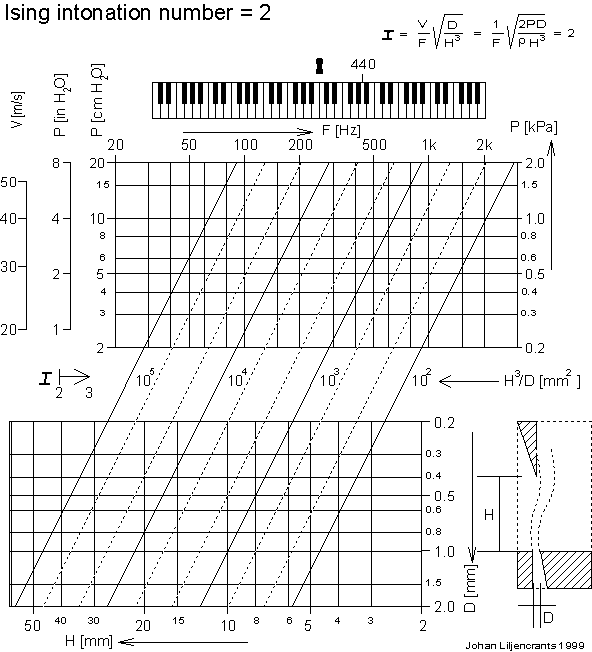
Intonation number

The dimensionless intonation number I was developed by Ising as part of his dissertation in modeling the wave motion of the flue jet under influence of the acoustic flow. It represents an elegant formulation of the trading relations between jet initial velocity V, jet initial thickness D, cut-up height H, and pipe fundamental frequency F. When I=2 the jet has optimal conditions to drive the pipe fundamental. The essence is that pipes of different size/frequency but with the same general design and intonation number will also have similar voice properties. It does not account for various details like shape of the flue channel, nicking, or beards and bridges.

The original formula is the left one in the figure, including the jet initial velocity V. For convenience my diagram instead uses the next alternative where V is (approximately) derived from the blowing pressure P, using the Bernoulli law.

How to use

Designing a pipe you would initially know pipe frequency F and pressure P. Mark the corresponding point in the upper rectangular diagram. Put a ruler through this point and parallel to the sloping lines, maybe draw a line. This line marks a constant value of H³/D and along it you can read corresponding pairs of values for H and D from the scales in the lower rectangular diagram. You can choose those within limited ranges, wide for D, narrow for H, all giving the optimal I=2. But be warned that this does not automatically mean the pipe will sound the same, for instance the (sound) power will obviously vary with D. And a change in H will require a minor adjustment in pipe length to keep F.

You can also work other directions. For instance, with an existing pipe you could locate H and D in the lower diagram and then follow the sloping lines into the upper. Here you can find the suitable pressure once you know the frequency.

Extra juggling

Normally you might want I to be somewhat larger than 2 in order to enhance higher harmonics, but near I=3 the pipe will overblow. Following the slopes from the upper to the lower diagram you can also add a sideways translation to account for a higher I. The arrow at left, marked I 2->3, tells how much is required to reach I=3.

The aerodynamically effective value of D can be somewhat smaller than the mechanical measure you find with a blade gauge. This is because of viscous friction boundary layer effects in the upstream flue channel. Pressure P is immediately upstream the flue and may differ from the main system pressure, for instance because of throttling in the pipe toe.

Reference

Ising, H: Erforschung und Planung des Orgelklanges. (In German; Exploration and planning of organ sound). Walcker Hausmitteilung Nr 42, Juni 1971, pp 38-57.

-----------------------------------------------------------------------------

(Author JLs 990409)